The Law of Total Trumps (or Tricks).
- geoff2959
- May 31, 2022
- 5 min read
Updated: Dec 17, 2024
How can a law that is wrong most of the time be so useful?
The law of total trumps (or the law of total tricks, both names are used) says that the total number of tricks that can be taken by North/South playing in their trump suit and by East/West playing in theirs (total tricks) is equal to the length of North/South's trump suit plus the length of East/West's trump suit (total trumps). For example, suppose North/South have a 4:4 spade fit, then they have 8 trumps. If East/West have a 5:3 heart fit then they also have 8 trumps making 16 'total trumps'. The law then states that the 'total tricks' will be equal 16. Note that this takes no account of the points held by the two sides!
With an equal point holding then we might expect each side to be able to take 8 tricks but this is not what the law says. It makes no statement about how the 16 tricks tricks are split between the two sides, only that the total will be 16. The relative point holdings and the positions of the cards determine the split.
For an example of the use of this law consider the following competitive auction:
North East South West
1H 1S 2H 2S
?
North can reason at this point that both sides have at least 8 trumps and therefore there are at least 16 total tricks. If East can only make 2 spades that's 8 tricks so applying the law says that North can also make 8 tricks playing in hearts. North could therefore bid 3 hearts since one off would be a good result against East making 2 spades. If, however, East can only make 7 tricks then North can make 9 and 3 hearts will make. By bidding 3 hearts, North would win either way! In fact, however, the way I play this is not North's job. A bid of 3 hearts by North here would show extra values such as an extra trump or a better than opening hand. With no extra values North should pass and if East passes it is South's job to perform this analysis and bid 3 hearts, even with no extra values! In this sort of auction, if you are non-vulnerable, then every expert will agree that it's alway right to bid one more. If you are vulnerable, however, then be a little circumspect since 1 down doubled is the dreaded -200 which is a bottom when East/West can't make game.
Are things really this simple? Does this law really hold? I decided to investigate it by using Deal 3.1 to deal 1000 random hands, perform double-dummy analysis on each one and see how often the law was right. Dealing the hands was simple since I just wanted random hands. There was a slight problem with the analysis since in about 8% of hands the number of tricks made depended upon which hand played the contract, so if the law worked in one case it didn't for the other. I didn't have any bidding to go on so I counted half for these deals. Even doing this, I found that the law was right only 40% of the time. It was wrong more often than it was right!
The total tricks for a hand varied from 14 to 22 over my sample of 1000 hands so I wondered if things might be better if I concentrated on the 'competitive area' from 16 to 18 total tricks, say, but the law was right on only 36% of these deals!
Thinking about this, I realised that this was because, even for a fixed number of total trumps, there was quite a range of possible outcomes and I was asking how often the law was exactly right. Perhaps what I should be asking was "Does the average number of total tricks agree with predictions?" To appreciate what I'm saying, suppose for example that I dealt 3000 hands and that I got 1000 hands where the law was right, 1000 where there was one more total tricks than predicted and 1000 where there was one less. The law would only be exactly right only one third of the time but, on average. its predictions would be absolutely correct. The 1000 overtricks would be cancelled by the 1000 undertricks!
I therefore decided to see, for each number of total trumps from 14 to 23, how well the average number of total tricks agreed with predictions. In my first 1000 random deals I had two or three hundred deals with 16 or 17 total trumps but very few deals with greater than 20. I therefore changed my simulations so that I analysed approximately 300 deals for each number of total tricks. My results are shown below
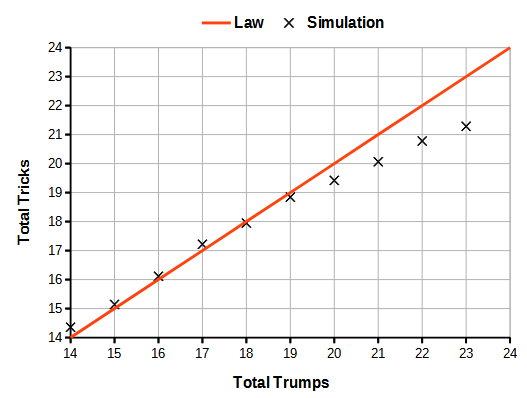
It can be seen that the average number of total tricks is well predicted up to about 19 total trumps, Indeed, at 18 total trumps, the law and the simulated results virtually coincide. At 20 total trumps, however, the law overpredicts by about half a trick on average and it is a full trick out at 21 total trumps.
We now begin to understand, perhaps, why the law works. Bridge is a game of chance, you can never make the right decision on every hand but what we aim to do is to win on average. This law works extremely well, on average, in a normal competitive situation with about 16 to 20 total tricks and hence it is well worth applying.
The distribution of total tricks for 14, 18 and 22 total trumps is shown below:
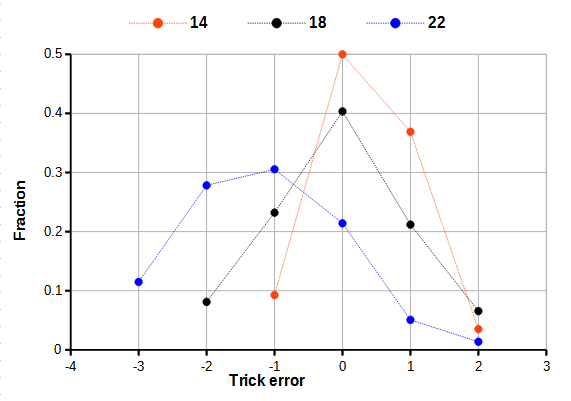
These graphs illustrate the width of the distribution and hence why the law is wrong most of the time. It can be seen that the law is most often correct (zero error) for 14 total trumps but even then it is only correct half the time. This is a rare situation since neither side can have an 8 card or greater trump fit and the law will usually be irrelevant when it occurs since such a hand will often be played in no trumps. The near perfect symmetry of the 18 total trumps result emphasises how well the law works on average for this case. By the time you are considering 22 total trumps, however, it is clear that the law is wrong on average and also that the range of error is much increased.
See my post "Should you prefer the four four fit over the five three?" for another example of the use of Deal 3.1. I'm happy to provide the code for these analyses or, if you have anything you would like me to investigate, contact me using the form below.
Commentaires